Overview
This session focuses on the work students do in the later grades in Investigations to support their understanding and develop fluency with addition and subtraction.
You will:
- estimate answers to addition and subtraction problems
- learn about the strategies students use to solve addition and subtraction problems
- see examples of students using addition and subtraction strategies and try some of the strategies yourself
- play games that connect the base-ten number system and addition and subtraction
- consider the underlying generalizations students rely on as they solve addition and subtraction problems
Getting Started: Estimation
In Investigations, students are often asked to estimate answers to addition and subtraction problems before they solve them. When students estimate, they use what they know about the operations and the place value of the numbers in a problem to determine the magnitude of their answer. Estimating answers to computation problems helps students determine the reasonableness of their answers to the problems, an important component of computational fluency.
Feature – Classroom Routines and Ten-Minute Math
Classroom Routines (K-2) and Ten-Minute Math activities (Gr. 3-5) are critical pieces of the review and practice that are built into Investigations. These activities, to be done daily outside the regular math class, provide practice with current concepts and skills or a review of previously introduced content.
Closest Estimate
The following Ten-Minute Math activity, Closest Estimate, appears in grades three through five. Students are asked to determine which out of three numbers is the closest estimate for a particular problem.
For each of the problems below:
- Look at the problem and the three possible estimates (In the classroom these problems are revealed for about 30 seconds)
- Decide which of the estimates you think is closest to the actual answer
Problem A
| 342 + 273 ≈ | 500 | 550 | 600 |
Problem B
| 435 - 119 ≈ | 200 | 350 | 300 |
Problem C
| 354 - 291 ≈ | 50 | 100 | 150 |
- Why do you think the one you chose is the closest estimate?
- Do you think your estimate is more or less than the actual answer? Why?
Activity 1: Place Value and Addition and Subtraction
In this activity, you will play Investigations games from Kindergarten through fourth grade that highlight the base-ten aspects of our number system; and consider what aspects of place value and the number system are practiced while playing these games.
A thorough understanding of the base-ten number system is one of the critical building blocks for developing computational fluency. In Investigations, students work on making sense of place value and the structure of the base-ten number system in many different activities that are interwoven with the work they do with addition and subtraction. As students engage in activities and games that highlight the base-ten aspects of our number system they see the usefulness and importance of place value in their computation. They can then apply their understanding to efficiently solve computation problems.
Play at least three of the following games. As you play, keep the following questions in mind:
- What aspects of place value and the number system does this game highlight?
- How do you think playing this game might help students solve addition and subtraction problems?
| K | How Many to 10? |
| 1st | Make 10 |
| 1st | Roll Tens |
| 2nd | Capture 5 |
| 2nd | Plus or Minus 10 or 100 |
| 3rd | Close to 100 |
| 3rd | How Far from 100? |
| 4th | Close to 1000 |
Consider:
- What aspects of place value and the number system do the games you played highlight?
- How might playing these games help students with solving addition and subtraction problems?
Activity 2: Addition
In this activity, you will determine how close the sum of an addition problem is to 100; learn about the addition strategies students develop from second to fifth grade; solve an addition problem using different strategies; and consider how addition strategies for whole numbers apply to addition with mixed numbers.
Today’s Number: More or Less
Today’s Number: More or Less? is a second grade Classroom Routine. In this routine, students think about whether the answer to an addition or subtraction problem is more or less than 50 (or 100 or 500). The class then determines the exact answer which is Today’s Number.
Answer the following questions for problems A, B, C.
- Is the sum more or less than 100?
- How did you decide?
- What did you pay attention to when you looked at these numbers?
- Did you use combinations you know that equal 100 to help you?
Problem A
Problem B
Problem C
Addition Strategies
In Session 1, you saw how first grade students solved addition and subtraction problems using counting all, counting on or back, counting or adding up (subtraction only) or numerical reasoning. As students gain a better understanding of the structure of the base-ten number system and the operations of addition and subtraction, they apply this knowledge to develop strategies for solving addition and subtraction problems with larger numbers. In second through fourth-grade students work on developing these strategies, comparing them and then becoming more efficient and flexible in using them.
Read Addition Strategies about the different categories of addition strategies students use to solve problems.
Kindergarten through fifth grade students solve addition and subtraction problems both in story problems and without contexts (in fifth grade the addition and subtraction problems students solve are with fractions and mixed numbers).
One of the contexts used for addition and subtraction in second and third grade is the Sticker Station. This context helps students keep in mind the hundreds, tens and ones structure of numbers and supports students' use of strategies that involve breaking apart numbers by place value to solve addition and subtraction problems.
Read the following Math Practice Note related to the Sticker Station.

Solve the problem mentally in any way that works for you.
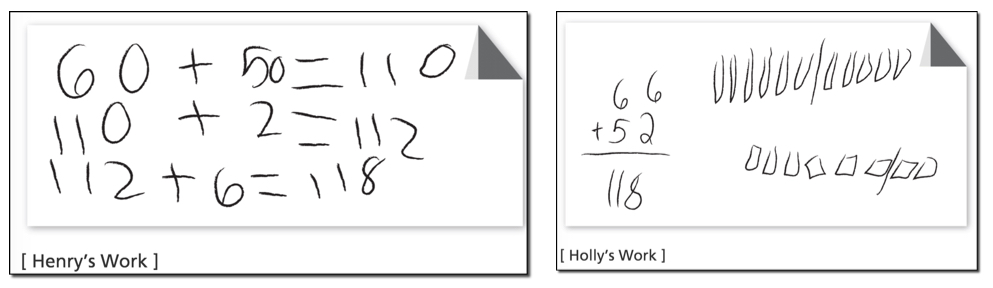
Franco had 66 car stickers. Jake gave him 52 car stickers.
How many car stickers does Franco have now?
It may not always be evident that there are similarities among students’ strategies. Their representations and how they break apart the numbers may be different. Allow students time to look at and compare their work with others.
Review the samples of second grade student work.
As you look at each piece of student work consider the following questions:
- Which strategy from the reading, Addition Strategies, did the students use?
- How did each student use the strategy differently?
66 + 52 Henry and Holly
66 + 52 Chen and Simon

66 + 52 Nadia and Juanita

Choose the work of a pair of students (Henry and Holly, Chen and Simon, or Nadia and Juanita). In the Addition Student Work Forum compare the pieces of student work and describe what you think the students understood to solve the problem in the way they did.
Use two of the strategies described in the reading, Addition Strategies, to solve the following problem:
248 + 337
Consider:
- What understandings of the base-ten number system and place value did you use in your solutions?
Students engage in Math Practice 7: Making use of structure as they apply what they know about the base-ten structure of our number system to solving addition and subtraction problems.
Read this third grade MP7 Mathematical Practice essay for examples of how students engage in MP7.
In what ways are the students in this essay making use of the structure of the base-ten number system?
Addition with Mixed Numbers
In fourth and fifth grade, students add and subtract fractions and mixed numbers. They use some of the same strategies they use for adding whole numbers to add mixed numbers.
Solve the following problem:
Venetta is filling up her fish tank. The fish tank has 1 2/3 gallons of water in it already. She adds 3 2/3 gallons of water to it. How many gallons of water are now in her fish tank?
Students in fourth grade solve this problem and discuss their strategies. Here are some possible solutions for the problem.
Feature - Students Might Say
Some discussions and activities include a “Student Might Say” section. These are examples of statements that students might make in response to questions by the teacher.


Students then discuss whether you can break up mixed numbers into parts when you add them. Click [show] to view some possible student responses.
How are these students’ strategies with mixed numbers similar to the strategies you read about in the reading, Addition Strategies?
Activity 3: Subtraction
In this activity, you will consider a variety of subtraction strategies by solving a subtraction problem using different first steps and examining student work for a subtraction problem.
Students in second through fourth grade develop, expand and refine their strategies for solving subtraction problems. The students’ strategies are based on their understanding of place value, the base-ten number system and the operations of subtraction and addition.
Starter Problems
Examining and comparing computation strategies supports students in developing a repertoire of strategies to solve addition and subtraction problems efficiently. In third, fourth and fifth-grade, students solve and discuss ‘Starter Problems’.
Mentally solve the set of problems below.
| 150 - 70 |
| 150 - 80 |
| 78 + 22 |
- Choose one problem from the set as your “first step” to solve the problem:
150 - 78
- Record the next steps of your solution to find the answer to 150 – 78.
- Repeat the process with a different starter problem as your “first step” to solve the problem.
Consider:
- What understandings of the base-ten number system and place value did you use in your solutions?
Watch and listen to three possible solutions to 150 –78 using the three starter problems as three different first steps to solve 150 – 78.
Subtraction Strategies
Read Subtraction Strategies which discusses the different types of strategies students use to solve subtraction problems.
Unmarked Number Line
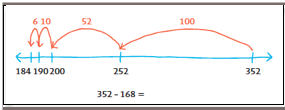
In Investigations students sometimes use unmarked number lines to solve and/or represent solutions to addition and subtraction problems. An unmarked number line is not marked to begin with, instead students just mark on the number line the numbers pertaining to their solution.
The solutions in the reading, Subtraction Strategies, were represented both in equations and on a number line.
How can using a number line to solve or represent a solution to an addition or subtraction problem be beneficial?

Read Mathematical Representations for Addition and Subtraction about the addition and subtraction representations students are introduced to in Investigations.
Solve the following problems using some of the strategies described in the reading, Subtraction Strategies. Use a number line to solve and/or represent at least one of your solutions.
| 42 - 17 |
| 924 - 496 |
| 1,435 - 619 |
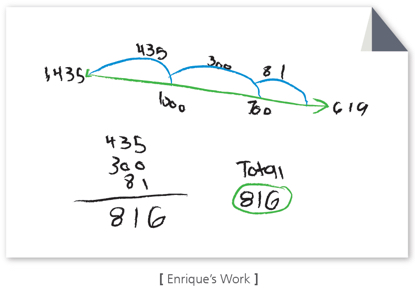
Look at the following pieces of student work for the problem, 1,435 – 619.
Consider:
- What strategy do you think the student used?
- What do you think the student needed to understand about place value, the number system and/or about subtraction to solve the problem in this way?
| 1,435 - 619 Steve | 1,435 - 619 Anna |
 |
 |
| 1,435 - 619 Enrique | 1,435 - 619 Ursula |
 |
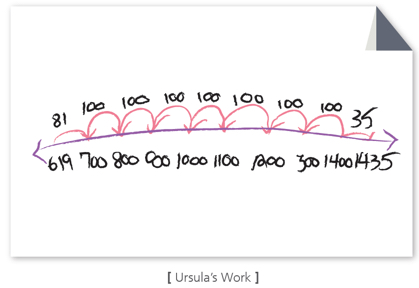 |
Click on Show to see how the students’ strategies can be categorized:
Lens on Equity
Students who have a strong sense of mathematical agency are: “active participants in, rather than passive recipients of, their mathematics education experiences.” (Aguirre, Mayfield-Ingram & Martin, 2013, p. 15)
In Investigations, students solve computation problems based on what they understand about numbers, the base-10 number system, and the operations, rather than being taught procedures they have to use. How might using strategies based in their own understanding, and choosing which strategies they use, have an effect on students’ sense of agency?
Discussion
Once you have completed the work in this session, go to the Session 3 Discussion Forum.
What understandings about numbers and the operations of addition and subtraction do students need to efficiently solve problems using the strategies discussed in this session?
Readings
- Addition Strategies from 4U5 - Large Numbers and Landmarks.
- Mathematical Practice 7 Essay from 3U3 - Travel Stories and Collections.
- Subtraction Strategies from 4U5 - Large Numbers and Landmarks.
- Mathematical Representations for Addition and Subtraction from 3U3 - Travel Stories and Collections.
The readings above are all published in Investigations in Number, Data, and Space®, 3rd ed. Northbrook, IL: Savvas Learning Company, LLC, 2017.
Key Learnings
- Estimating answers to computation problems helps students determine the reasonableness of their answers to the problems, an important component of computational fluency.
- When students estimate, they use what they know about the operations and the place value of the numbers in a problem to determine the magnitude of their answer.
- As students play games and solve problems that focus on the base-ten number system, place value and addition and subtraction they see the usefulness and importance of place value in their computation. They apply their understanding of place value and the number system to efficiently solve computation problems.
- The strategies students use are based on their understanding of place value, the base-ten number system and the operations of subtraction and addition.
- Describing, analyzing, and comparing strategies is an important part of the work students do in grades three, four, and five in order to develop strategies they can use efficiently and flexibly.
- Students rely on generalizations based on what they understand about numbers and the operations to solve problems.

